Bodies which orbit a large mass move at velocities which depend on their distance from that mass. In the animation at the right, you can see two planets orbiting the sun. The planet closest to the sun moves faster than the planet further away. The velocity of the orbiting body does not increase linearly; the relationship is such that the velocity increases inversely as the square root of the orbital radius: 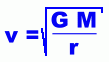where G is the gravitational constant, and M is the central mass. For example, if one planet were 16 times farther from the sun than another, its velocity would be one quarter of the other planet's. The important principle here is that as an object moves closer to the body it is orbiting around, its velocity increases. 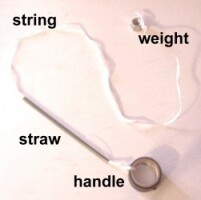Here is a simple demonstration you can use to illustrate this fact. You'll need a metre or two of string, a strong plastic straw, a weight of some sort, and a handle. We used an old roll of tape for a handle, and a large nut for a weight. 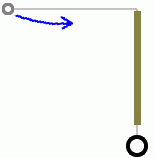The aim is to spin the weight in a circle, while holding the straw in one hand and the handle with the other. By holding the handle in position near the bottom of the straw, you can make the weight maintain a steady circular orbit. Be careful: you might consider using a weight that won't cause too much damage if it hits you in the head. We overlooked this danger, and paid the price! 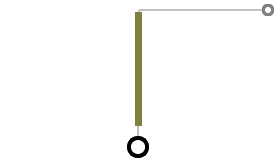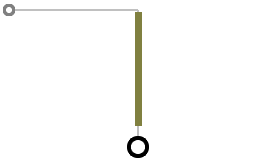This is what should happen after you get the weight spinning. You will feel a strong force pulling the handle upwards, which you must counteract with a force of your own, to maintain the circular orbit. The force you apply is analagous to the gravitational force. This demonstration all by itself will let you calculate the velocity of the orbiting weight. Measure the radius of the circular path, work out the circumference, and divide this by the time for one orbit. (The latter value can be obtained by timing 20 orbits and dividing by 20) Now pull down on the handle. Keep pulling until the weight has moved inwards, and is moving considerably faster. This clearly illustrates that a shorter radius of orbit results in a higher velocity. Once again you could calculate the velocity of the moving weight. In fact, you can make a very nice graph of velocity vs radius, by calculating and measuring both values for a series of different radii. What do you think the graph would look like? Mathematically, you could then describe the relationship between   v and  r, using the regression menu on your TI83+ calculator. This little demonstration can lead to a number of interesting mathematical explorations! Resources